Topological resonance in Weyl semimetals in a circularly polarized optical pulse

摘要

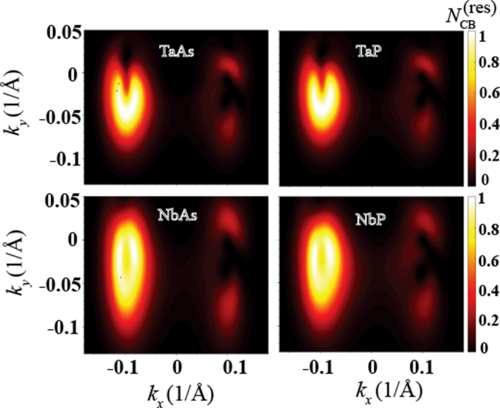
We study theoretically the ultrafast electron dynamics of three-dimensional Weyl semimetals in the field of a laser pulse. For a circularly polarized pulse, such dynamics is governed by topological resonance, which manifests itself as a specific conduction band population distribution in the vicinity of the Weyl points. The topological resonance is determined by the competition between the topological phase and the dynamic phase and depends on the handedness of a circularly polarized pulse. Also, we show that the conduction band population induced by a circularly polarized pulse that consists of two oscillations with opposite handedness is highly chiral, which represents the intrinsic chirality of the Weyl points.rocessing, and biomedical detection and sensing with chirality resolution.